Trig Antiderivatives Chart
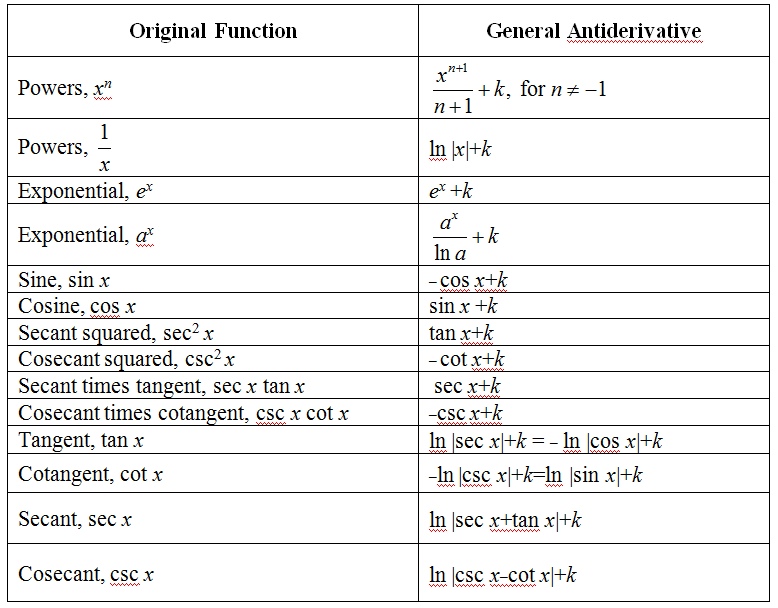
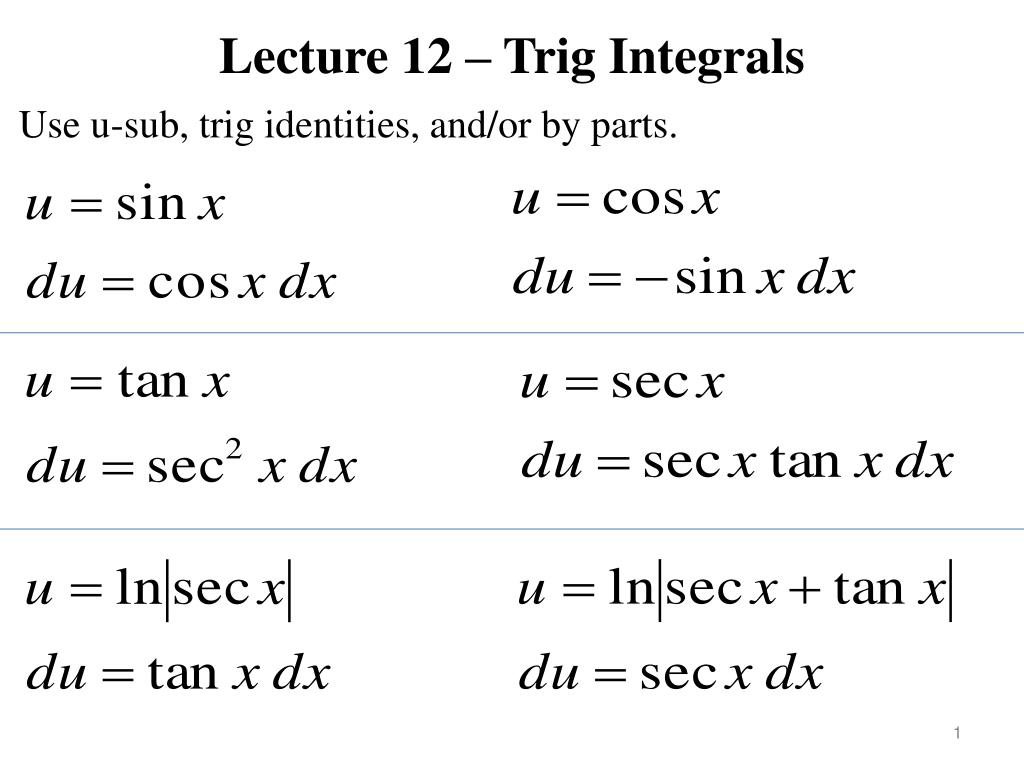
Trig Antiderivatives Chart - We will learn some techniques but it is in general not possible to give anti derivatives for a function, if it looks simple. State the power rule for integrals. Parts, partial fractions, trig substitution, etc. Depending upon your instructor, you may be expected to memorize these antiderivatives. Web the following is a list of integrals ( antiderivative functions) of trigonometric functions. Find the general antiderivative of a given function. Complicated functions can be computed from these using techniques like. For a longer list of antiderivative formulas, see your textbook. On other occasions, some manipulation will be needed first. F (x) = xn xn+1 (if n 6= 1) f(x) = n+1 + c. Explain the terms and notation used for an indefinite integral. $\int \cos x\ dx = \sin x + c$. (you will obtain them in the exercises.) integral rule. Two of the derivatives will be derived. Web functions involving trigonometric functions are useful as they are good at describing periodic behavior. Web list of integrals of trigonometric functions. (you will obtain them in the exercises.) integral rule. Explain the terms and notation used for an indefinite integral. Web those would be derivatives, definite integrals, and antiderivatives (now also called indefinite integrals). Web the table below shows you how to differentiate and integrate 18 of the most common functions. Explain the terms and notation used for an indefinite integral. We just need to use the rule \begin{gather*} \text{if } f(x) = x^n \text{ then } f(x) = \frac{1}{n+1} x^{n+1} + c. When you learn about the fundamental theorem of calculus, you will learn that the antiderivative has a very, very important property. Here we introduce notation for. Find the. $\int \cos (ax+b) dx = \frac {1} {a} \sin (ax+b) + c$. Here we introduce notation for. For a longer list of antiderivative formulas, see your textbook. If the power n of cosine is odd (n = 2k + 1), save one cosine factor and use cos2(x) = 1 express the rest of the factors in terms of sine: This. Web functions involving trigonometric functions are useful as they are good at describing periodic behavior. We just need to use the rule \begin{gather*} \text{if } f(x) = x^n \text{ then } f(x) = \frac{1}{n+1} x^{n+1} + c. F (x) = k (k is constant) f(x) = kx + c. For antiderivatives involving both exponential and trigonometric functions, see list of. Explain the terms and notation used for an indefinite integral. State the power rule for integrals. Web we’ll start this process off by taking a look at the derivatives of the six trig functions. For antiderivatives involving both exponential and trigonometric functions, see list of integrals of exponential functions. On other occasions, some manipulation will be needed first. For a complete list of antiderivative functions, see lists of integrals. (you will obtain them in the exercises.) integral rule. Depending upon your instructor, you may be expected to memorize these antiderivatives. If the power n of cosine is odd (n = 2k + 1), save one cosine factor and use cos2(x) = 1 express the rest of the factors. Web table of antiderivatives of basic functions (table 7.2.1 in the textbook) function antiderivative. For a longer list of antiderivative formulas, see your textbook. Web in the video, we work out the antiderivatives of the four remaining trig functions. Web finding antiderivatives of polynomials is generally not too hard. For antiderivatives involving both exponential and trigonometric functions, see list of. State the power rule for integrals. State the power rule for integrals. $\int \cos x\ dx = \sin x + c$. For antiderivatives involving both exponential and trigonometric functions, see list of integrals of exponential functions. Depending upon your instructor, you may be expected to memorize these antiderivatives. Web not to keep you in suspense, here are the antiderivatives of all six trigonometric functions. Find the general antiderivative of a given function. F (x) = xn xn+1 (if n 6= 1) f(x) = n+1 + c. Web trigonometric derivatives and integrals. Sometimes, it may be possible to use one of these standard forms directly. For antiderivatives involving both exponential and trigonometric functions, see list of integrals of exponential functions. Parts, partial fractions, trig substitution, etc. $\int \cos x\ dx = \sin x + c$. Web list of integrals of trigonometric functions. We are integrating, we need to be able to recognise standard forms. F (x) = k (k is constant) f(x) = kx + c. Web list of derivatives of trig & inverse trig functions. Given a function \(f\), we use the notation \(f′(x)\) or \(\dfrac{df}{dx}\) to denote the derivative of \(f\). Explain the terms and notation used for an indefinite integral. Here we introduce notation for. It is assumed that you are familiar with the following rules of differentiation. Web not to keep you in suspense, here are the antiderivatives of all six trigonometric functions. Web these rules can be used for the antidifferentiation of algebraic functions, exponential function, trigonometric functions, hyperbolic functions, logarithmic function, and constant function. For a complete list of antiderivative functions, see lists of integrals. We just need to use the rule \begin{gather*} \text{if } f(x) = x^n \text{ then } f(x) = \frac{1}{n+1} x^{n+1} + c. Two of the derivatives will be derived.
6.8 Finding Antiderivatives and Indefinite Integrals (Part 5

Basic Antiderivatives of Trigonometric Functions YouTube

Antiderivative Rules List, Formulas, Examples What are
Common Trig Derivatives And Integrals Slidesharetrick 302

4.82 Some trigonometric antiderivatives YouTube
Trig Antiderivatives

Common Trig Derivatives And Integrals Slidesharetrick 302

6.1b Antiderivatives of Trig Functions AP Calculus YouTube

Antiderivatives of Trig functions YouTube
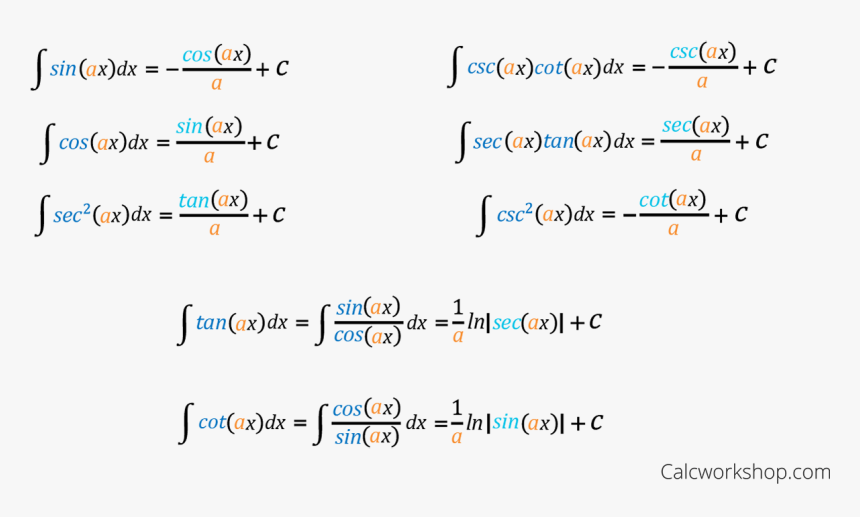
Rules For Integrating Trig Functions Antiderivative Trig Chart, HD
Web We’ll Start This Process Off By Taking A Look At The Derivatives Of The Six Trig Functions.
Web Find The Derivatives Of The Standard Trigonometric Functions.
Let Us Go Through The Important Antiderivative Rules In The Sections Below.
One Of The Most Important Types Of Motion In Physics Is Simple Harmonic Motion, Which Is Associated With Such Systems As An Object With Mass Oscillating On A Spring.
Related Post: